Penrose Tiling 2: Implementation and Examples
Posted by christian on 26 May 2015
This article demonstrates the use of the Penrose tiling class described in the previous blog post.
The code described in this post is available under the GPL licence on GitHub.
Example 1: Inflation of a single tile
The Initial triangle is defined with the origin at the centre of its base:

An initial Robinson triangle for inflation to generate a Penrose tiling
This initial triangle is inflated for five generations. The default configuration is used for its appearance.
# example1.py
import math
from penrose import PenroseP3, BtileL, psi
# A simple example starting with a BL tile
scale = 100
tiling = PenroseP3(scale, ngen=5)
theta = 2*math.pi / 5
rot = math.cos(theta) + 1j*math.sin(theta)
A = -scale/2 + 0j
B = scale/2 * rot
C = scale/2 / psi + 0j
tiling.set_initial_tiles([BtileL(A, B, C)])
tiling.make_tiling()
tiling.write_svg('example1.svg')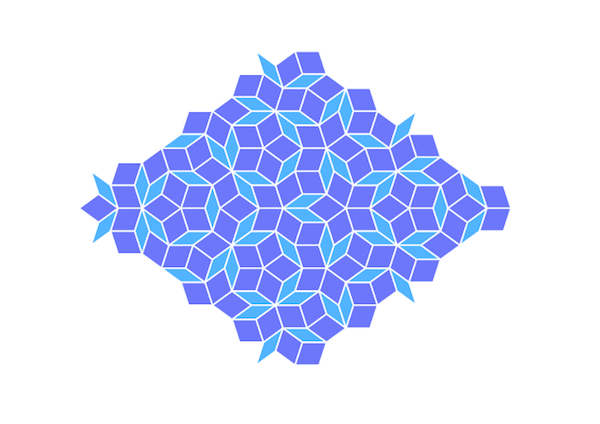
Example 1: A P3 Penrose tiling after inflation of the $B_L$ triangle five times
Example 2: A "sun" initialized by a semicircular arrangement of $B_S$ tiles.
The five initial BS tiles are arranged as below, with their vertices calculated by successive rotations of the edge BA1.

The initial configuration of five $B_S$ tiles for inflation into a P3 Penrose tiling
# example2.py
import math
from penrose import PenroseP3, BtileS, BtileL
# A "sun"
scale = 100
config={'tile-opacity': 0.9, 'stroke-colour': '#800',
'Stile-colour': '#f00', 'Ltile-colour': '#ff0'}
tiling = PenroseP3(scale*1.1, ngen=4, config=config)
theta = math.pi / 5
alpha = math.cos(theta)
rot = math.cos(theta) + 1j*math.sin(theta)
A1 = scale + 0.j
B = 0 + 0j
C1 = C2 = A1 * rot
A2 = A3 = C1 * rot
C3 = C4 = A3 * rot
A4 = A5 = C4 * rot
C5 = -A1
tiling.set_initial_tiles([BtileS(A1, B, C1), BtileS(A2, B, C2),
BtileS(A3, B, C3), BtileS(A4, B, C4),
BtileS(A5, B, C5)])
tiling.make_tiling()
tiling.write_svg('example2.svg')
A P3 Penrose tiling after inflation of an initial arrangement of five $B_S$ triangles
Example 3: A star, including coloured arc lines
The five initial tiles are laid out as below, with the vertices p, q, r and s calculated by rotation of the edges AB1 and AC5.

The initial configuration of Robinson triangles for inflation into a star-shaped Penrose tiling
The completed star is rotated by 90 degrees so that one of its vertices points upwards and the arc and tile colours customized.
# example3.py
import math
from penrose import PenroseP3, BtileL, psi
# A star with five-fold symmetry
# The Golden ratio
phi = 1 / psi
scale = 100
config = {'draw-arcs': True,
'Aarc-colour': '#ff5e25',
'Carc-colour': 'none',
'Stile-colour': '#090',
'Ltile-colour': '#9f3',
'rotate': math.pi/2}
tiling = PenroseP3(scale*2, ngen=5, config=config)
theta = 2*math.pi / 5
rot = math.cos(theta) + 1j*math.sin(theta)
B1 = scale
p = B1 * rot
q = p*rot
C5 = -scale * phi
r = C5 / rot
s = r / rot
A = [0]*5
B = [scale, p, p, q, q]
C = [s, s, r, r, C5]
tiling.set_initial_tiles([BtileL(*v) for v in zip(A, B, C)])
tiling.make_tiling()
tiling.write_svg('example3.svg')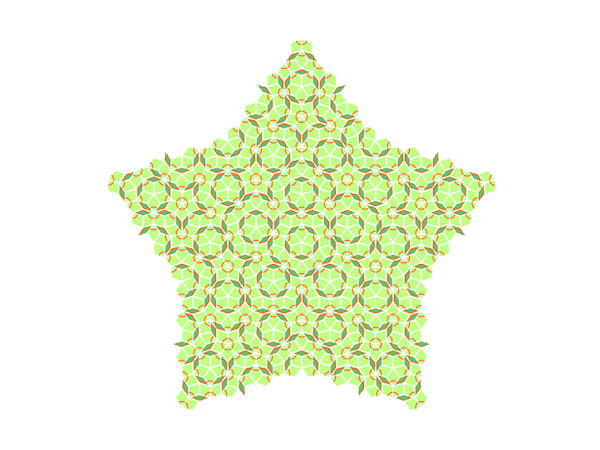
A P3 Penrose tiling in the shape of a five-pointed star